
There are also other ways of describing everything about a parabola, but this is often one of the simplest ways of doing so. To completely describe any parabola, all someone needs to know is: its dilation factor and the coordinates of its vertex. These three values, a, h, and k, will describe a unique parabola. This forces the y-coordinate of the vertex to become k. When (at the vertex), the entire squared term will always equal zero, and the result of the equation must equal k.
#VERTEX FORM OF A QUADRATIC HOW TO#
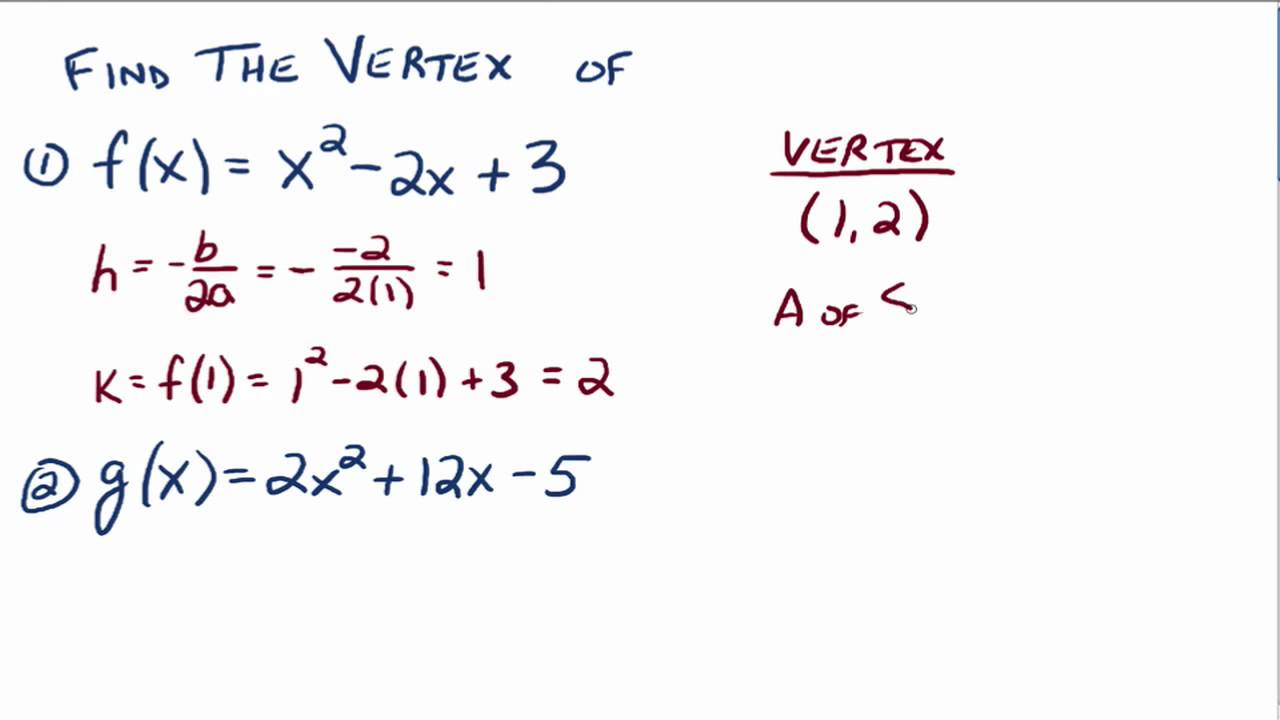
The vertex (h, k) is located at h b 2a, k f(h) f( b 2a) How To Given a graph of a quadratic function, write the equation of the function in general form. K determines the y-coordinate of the graph's vertex. The standard form of a quadratic function is f(x) a(x h)2 + k where a 0. For all values of x other than h, the squared quantity in parentheses must produce a value greater than zero (higher than the vertex). The table shows the linear and quadratic parent functions. In a quadratic function, the variable is always squared. A quadratic function is a function that can be written in the form of. Therefore, when a is positive, h becomes the x-coordinate at which the graph must reach its lowest point: its vertex. In Chapters 2 and 3, you studied linear functions of the form f(x) mx + b. Note that in the equation shown on the graph, when x is equal to h, the value in parentheses must equal zero, which is the smallest value that any squared real quantity can assume. What is the factored form of a quadratic function The.
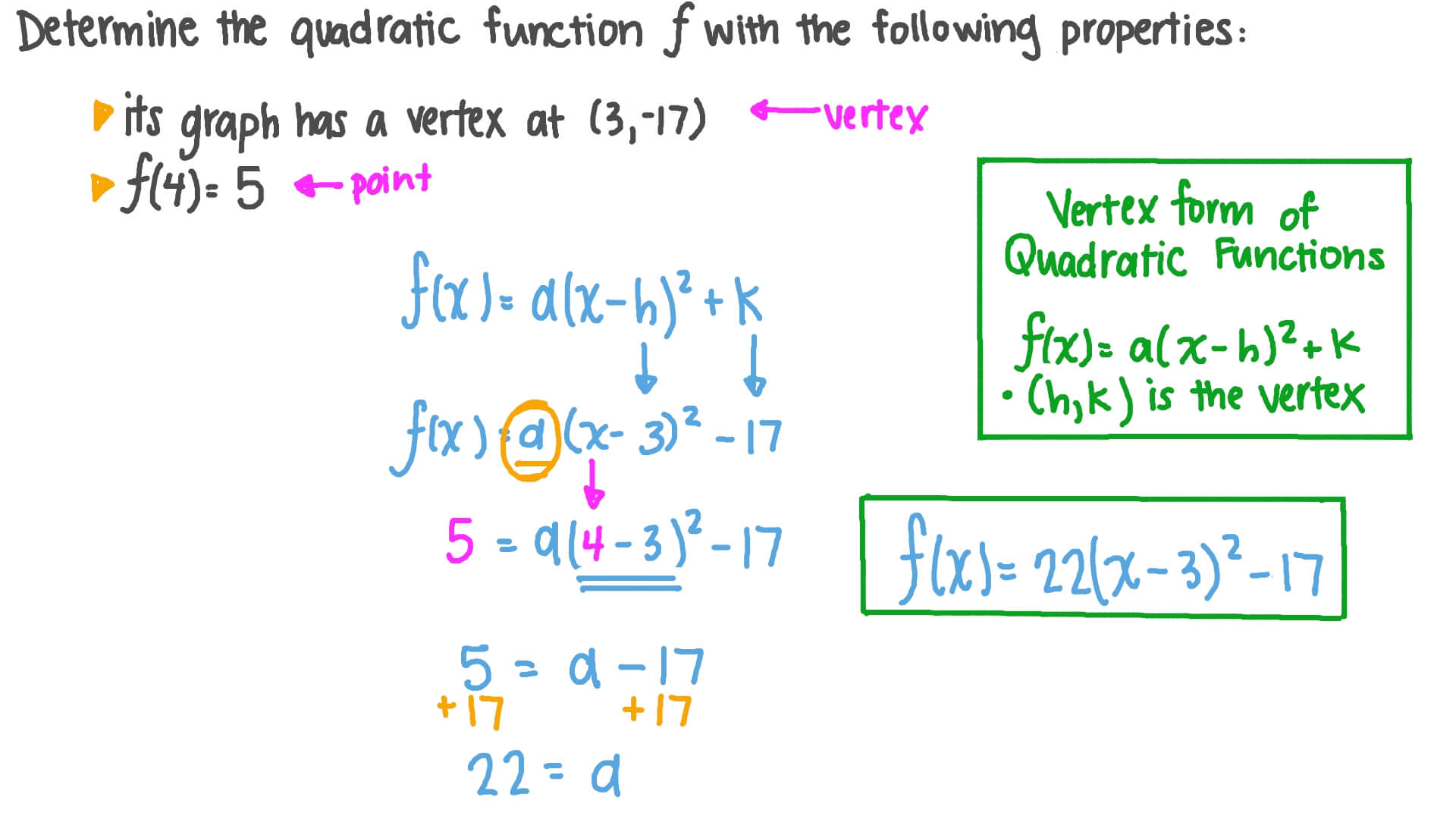
H determines the x-coordinate of the graph's vertex. The vertex form of a quadratic function is expressed as: ya(x-h)2+k, where a, h, and k are constants. Note what happens to the graph when you set a to a negative value. It determines how much the graph is stretched away from, or compressed towards, the x-axis. The x and y values simply represent different points that lie on the parabola. the vertex of the graph (the blue point labelled V) is moved on top of the other blue point on the graph: (-3, -1)Ī is referred to as the "dilation factor". Vertex Form The a value tells us the direction of the parabolas opening. It gets its name from the fact that we can easily see the vertex of the quadratic by. any part of the graph passes through the other blue point on the graph (-3, -1) This form that we get from completing the square is called vertex form. the graph becomes a horizontal line, or opens down A quadratic function is one of the form f(x) ax2 + bx + c, where a, b, and c are numbers with a not equal to zero. the vertex lies above, or below, the x-axis the vertex lies to the right, or left, of the y-axis Once you understand the effect that each slider has, see if you can adjust the sliders so that:


 0 kommentar(er)
0 kommentar(er)
